Question

Ajani is trying to experimentally measure Planck's constant h. He does this by shining different wavelengths of monochro- matic (i.e., single-wavelength) EM radiation on a metal plate with unknown work function W, and then measuring the stop- ping voltage required to bring the ejected electrons to a halt. When he uses λ1= 400 nm, he finds that a stopping voltage of V1 = 0.7 V is required. When he uses λ2 = 500 nm, he finds that a stopping voltage of V2= 0.2 V is required. Based on these two data points, what is Ajani's measurement of Planck's constant?

Answer 1

h = 1.01 x 10⁻³⁴ J.s

Step-by-step explanation:

The energy applied by the voltage must be equal to the energy associated with the wavelength of light:

`eV = (hc)/(\lambda)\\`

where,

e = charge on electron = 1.6 x 10⁻¹⁹ C

V = stopping potential

h = Planck's Constant = ?

c = speed of light = 3 x 10⁸ m/s

λ = wavelength of light

For λ = 400 nm = 4 x 10⁻⁷ m, V = 0.7 V:

`(1.6\ x\ 10^(-19)\ C)(0.7\ V) = (h(3\ x\ 10^8\ m/s))/(4\ x\ 10^(-7)\ m)\\`

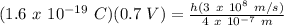
h = 1.49 x 10⁻³⁴ J.s

For λ = 500 nm = 5 x 10⁻⁷ m, V = 0.2 V:

`(1.6\ x\ 10^(-19)\ C)(0.2\ V) = (h(3\ x\ 10^8\ m/s))/(5\ x\ 10^(-7)\ m)\\`

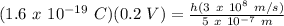
h = 0.53 x 10⁻³⁴ J.s

Taking average of both values:

`h = ((0.53+1.49)\ x\ 10^(-19)\ J.s)/(2)`

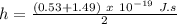
h = 1.01 x 10⁻³⁴ J.s