Question

A gas in a sealed container has a pressure of 125 atm at a temperature of 303 K. If the pressure in the container is increased to 200 atm, what is the new temperature if the volume remains constant?

(Show work pls :)!)

Answer 1

`T_2=484.8K`

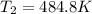
Step-by-step explanation:

Hello there!

In this case, according to the the variable temperature and pressure and constant volume, it turns out possible for us to calculate the new temperature via the Gay-Lussac's law as shown below:

`(T_2)/(P_2) =(T_1)/(P_1)`

Thus, by solving for the final temperature, T2, we obtain:

`T_2 =(T_1P_2)/(P_1)`

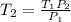
So we plug in the given data to obtain:

`T_2 =(303K*200atm)/(125atm)\\\\T_2=484.8K`

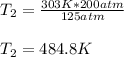
Best regards!