Answer:
99% confidence interval to estimate the mean discharge time for all capacitors of this type
(7.2146, 7.2854)
Explanation:
Step(i):-
Given that the sample size 'n' = 100
Given that the mean of sample x⁻ = 7.25 seconds
Given that the standard deviation of the sample (S) = 0.15 seconds
Level of significance = 0.01
Degrees of freedom = n-1 =100-1 =99
t₀.₀₁ , ₉₉ = 2.3646
We have to find that the 99% of the confidence interval of the mean
Step(ii):-
99% confidence interval to estimate the mean discharge time for all capacitors
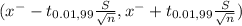
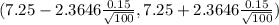
(7.25 - 0.0354 , 7.25 + 0.0354)
(7.2146, 7.2854)
Final answer:-
99% confidence interval to estimate the mean discharge time for all capacitors of this type
(7.2146, 7.2854)