A. square root property
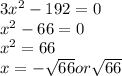
it has one value with x which is x^2 and it cn be easily solved without having to factorise, quadratic formula cant be used as it need ax^2+bx+c=0 format
B. factorising
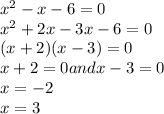
i just felt like this was easier to factorise than the other 2 options left
C. Completing the square

same reason personal preference
D- Quadratic
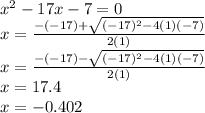
the 17 kinda threw me off and i didnt wanna get on factorising or doing completing the square so quadratic formal