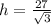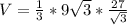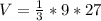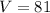Question

Pyramid A has a triangular base where each side measures 4 units and a volume of 36 cubic units. Pyramid B has the same height, but each side of its base is 6 units long.

Answer 1

81

Explanation:

Answer 2

The volume of pyramid B is 81 cubic units

Explanation:

Given

Pyramid A

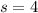`s = 4` -- base sides

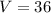`V = 36` -- Volume

Pyramid B

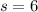`s = 6` --- base sides

Required

Determine the volume of pyramid B [Missing from the question]

From the question, we understand that both pyramids are equilateral triangular pyramids.

The volume is calculated as:

`V = (1)/(3) * B * h`

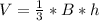
Where B represents the area of the base equilateral triangle, and it is calculated as:

`B = (1)/(2) * s^2 * sin(60)`

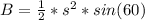
Where s represents the side lengths

First, we calculate the height of pyramid A

For Pyramid A, the base area is:

`B = (1)/(2) * s^2 * sin(60)`

`B = (1)/(2) * 4^2 * (\sqrt 3)/(2)`

`B = (1)/(2) * 16 * (\sqrt 3)/(2)`

`B = 4\sqrt 3`

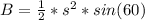
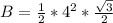
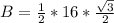
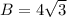
The height is calculated from:

`V = (1)/(3) * B * h`

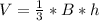
This gives:

`36 = (1)/(3) * 4\sqrt 3 * h`

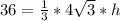
Make h the subject

`h = (3 * 36)/(4\sqrt 3)`

`h = (3 * 9)/(\sqrt 3)`

`h = (27)/(\sqrt 3)`

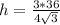
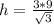
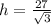
To calculate the volume of pyramid B, we make use of:

`V = (1)/(3) * B * h`

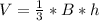
Since the heights of both pyramids are the same, we can make use of:

`h = (27)/(\sqrt 3)`

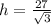
The base area B, is then calculated as:

`B = (1)/(2) * s^2 * sin(60)`

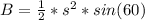
Where

`s = 6`

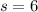
So:

`B = (1)/(2) * 6^2 * sin(60)`

`B = (1)/(2) * 36 * (\sqrt 3)/(2)`

`B = 9\sqrt 3`

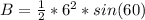
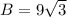
So:

`V = (1)/(3) * B * h`

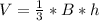
Where

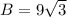`B = 9\sqrt 3` and
`h = (27)/(\sqrt 3)`

`V = (1)/(3) * 9\sqrt 3 * (27)/(\sqrt 3)`

`V = (1)/(3) * 9 * 27`

`V = 81`