Question

A third candle, in the shape of a right circular cone, has a volume of 16 cubic inches and a radius of 1.5 inches. What is the height, in inches, of the candle? Round your answer to the nearest inch.

Answer 1

Given:

Volume of a right circular cone = 16 cubic inches

Radius of the cone = 1.5 inches.

To find:

The height of the cone.

Solution:

The volume of a cone is:

`V=(1)/(3)\pi r^2h`

Where, r is the radius and h is the height of the cone.

Putting
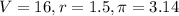`V=16,r=1.5,\pi=3.14` in the above formula.

`16=(1)/(3)(3.14)(1.5)^2h`

`48=(3.14)(2.25)h`

`48=7.065h`

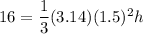
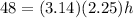
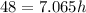
Divide both sides by 7.065.

`(48)/(7.065)=h`

`6.794=h`

`h\approx 7`

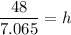
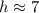
Therefore, the height of the cone is 7 inches.