Given Information :
- Length of parallel sides = 60 ft and 40 ft
- Height of the trapezoid = 30 ft
To calculate :
Calculation :
As we know that,
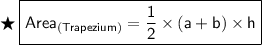
- a and b are length of parallel sides.
- h denotes height.
Substituting values, we get :
 Area =
Area =
 × ( 60 + 40 ) × 30 ft
× ( 60 + 40 ) × 30 ft

 Area =
Area =
 × 100 × 30 ft
× 100 × 30 ft

 Area = 1 × 100 × 15 ft
Area = 1 × 100 × 15 ft

 Area = 100 × 15 ft
Area = 100 × 15 ft

 Area = 1500 ft
Area = 1500 ft

Therefore,
- Area of the trapezoid is 1500 ft
