Given:
The length of the rectangle =

Width of the rectangle =
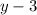
To find:
How much longer is the length?
Solution:
We need to find the difference between length and width of the rectangle.
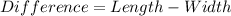

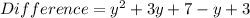

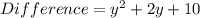
Therefore, the length of the rectangle is
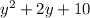 much longer than the width.
much longer than the width.