Question

An investigator compares the durability of two different compounds used in the manufacture of a certain automobile brake lining. A sample of 212 brakes using Compound 1 yields an average brake life of 47,895 miles. A sample of 180 brakes using Compound 2 yields an average brake life of 49,767 miles. Assume that the population standard deviation for Compound 1 is 1590 miles, while the population standard deviation for Compound 2 is 4152 miles.

1. Determine the 90% confidence interval for the true difference between average lifetimes for brakes using Compound 1 and brakes using Compound 2.
2. Find the point estimate for the true difference between the population means.

Answer 1

1. The 90% confidence interval for the true difference between average lifetimes for brakes using Compound 1 and brakes using Compound 2 is (-2411.84, -1332.16).

2. The point estimate for the true difference between the population means is of -1872.

Explanation:

Before building the confidence interval, we need to understand the central limit theorem and subtraction between normal variables.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

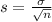For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

Subtraction of Normal Variables:

When two normal variables are subtracted, the mean is the subtraction of the means while the standard deviation is the square root of the sum of the variances.

A sample of 212 brakes using Compound 1 yields an average brake life of 47,895 miles. The population standard deviation for Compound 1 is 1590 miles.

This means that
`\mu_1 = 47895, \sigma_1 = 1590, n = 212, s_1 = (1590)/(√(212)) = 109.2`

A sample of 180 brakes using Compound 2 yields an average brake life of 49,767 miles. The population standard deviation for Compound 2 is 4152 miles.

This means that
`\mu_2 = 49767, \sigma_2 = 4152, n = 180, s_2 = (4152)/(√(180)) = 309.47`

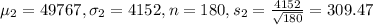
True difference between average lifetimes for brakes using Compound 1 and brakes using Compound 2.

This is the distribution 1 - 2. So

`\mu = \mu_1 - \mu_2 = 47895 - 49767 = -1872`

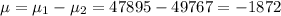
This is also the point estimate for the true difference between the population means, which is question 2.

`s = √(s_1^2+s_2^2) = √(109.2^2+309.47^2) = 328.17`

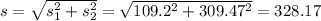
90% confidence interval

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

`\alpha = (1 - 0.9)/(2) = 0.05`

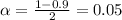
Now, we have to find z in the Ztable as such z has a pvalue of
`1 - \alpha`.

That is z with a pvalue of
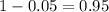`1 - 0.05 = 0.95`, so Z = 1.645.

Now, find the margin of error M as such

`M = zs = 1.645*328.17 = 539.84`

The lower end of the interval is the sample mean subtracted by M. So it is -1872 - 539.84 = -2411.84

The upper end of the interval is the sample mean added to M. So it is -1872 + 539.84 = -1332.16.

The 90% confidence interval for the true difference between average lifetimes for brakes using Compound 1 and brakes using Compound 2 is (-2411.84, -1332.16).