Question

A student researcher compares the ages of cars owned by students and cars owned by faculty at a local state college. A sample of 263 cars owned by students had an average age of 7.25 years. A sample of 291 cars owned by faculty had an average age of 7.12 years. Assume that the population standard deviation for cars owned by students is 3.77 years, while the population standard deviation for cars owned by faculty is 2.99 years. Determine the 90% confidence interval for the difference between the true mean ages for cars owned by students and faculty. Step 1 of 3: Find the point estimate for the true difference between the population means.

Answer 1

The point estimate for the true difference between the population means is 0.13.

The 90% confidence interval for the difference between the true mean ages for cars owned by students and faculty is between -0.35 years and 0.61 years.

Explanation:

To solve this question, before building the confidence interval, we need to understand the central limit theorem and subtraction between normal variables.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

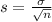For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

Subtraction between normal variables:

When we subtract two normal variables, the mean is the subtraction of the means while the standard deviation is the square root of the sum of the variances.

A sample of 263 cars owned by students had an average age of 7.25 years. The population standard deviation for cars owned by students is 3.77 years.

This means that:

`\mu_s = 7.25, \sigma_s = 3.77, n = 263, s_s = (3.77)/(√(263)) = 0.2325`

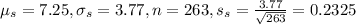
A sample of 291 cars owned by faculty had an average age of 7.12 years. The population standard deviation for cars owned by faculty is 2.99 years.

This means that:

`\mu_f = 7.12, \sigma_f = 2.99, n = 291, s_f = (2.99)/(√(291)) = 0.1753`

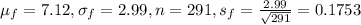
Difference between the true mean ages for cars owned by students and faculty.

Distribution s - f. So

`\mu = \mu_s - \mu_f = 7.25 - 7.12 = 0.13`

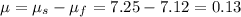
This is also the point estimate for the true difference between the population means.

`s = √(s_s^2+s_f^2) = √(0.2325^2+0.1753^2) = 0.2912`

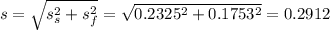
90% confidence interval for the difference:

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

`\alpha = (1 - 0.9)/(2) = 0.05`

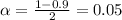
Now, we have to find z in the Ztable as such z has a pvalue of
`1 - \alpha`.

That is z with a pvalue of
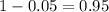`1 - 0.05 = 0.95`, so Z = 1.645.

Now, find the margin of error M as such

`M = zs = 1.645*0.2912 = 0.48`

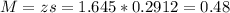
The lower end of the interval is the sample mean subtracted by M. So it is 0.13 - 0.48 = -0.35 years

The upper end of the interval is the sample mean added to M. So it is 0.13 + 0.48 = 0.61 years.

The 90% confidence interval for the difference between the true mean ages for cars owned by students and faculty is between -0.35 years and 0.61 years.