Answer:
Pvalue of 0.0446 < 0.05, which means that we reject the null hypothesis and accept the alternative hypothesis, that the true mean is larger than the specification.
Explanation:
The average weight of a package of rolled oats is supposed to be at most 18 ounces.
This means that the null hypothesis is:
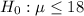
Is the true mean larger than the specification?
Due to the question asked, the alternate hypothesis is:
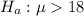
The test statistic is:

In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
18 is tested at the null hypothesis:
This means that
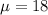
A sample of 18 packages shows a mean of 18.20 ounces with a sample standard deviation of 0.50 ounces.
This means that

Value of the test statistic:

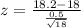
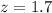
Pvalue of the test:
Probability of finding a sample mean above 18.2, which is 1 subtracted by the pvalue of z = 1.7.
Looking at the z-table, z = 1.7 has a pvalue of 0.9554.
1 - 0.9554 = 0.0446
0.0446 < 0.05, which means that we reject the null hypothesis and accept the alternative hypothesis, that the true mean is larger than the specification.