Question

A hammer strikes one end of a thick iron rail of length 8.80 m. A microphone located at the opposite end of the rail detects two pulses of sound, one that travels through the air and a longitudinal wave that travels through the rail. (The speeds of sound in air and in iron are 343 m/s and 5950 m/s, respectively.)

Required:
Find the separation in time between the arrivals of the two pulses.

Answer 1

ΔT = 0.02412 s

Step-by-step explanation:

We will simply calculate the time for both the waves to travel through rail distance.

FOR THE TRAVELING THROUGH RAIL:

`T_(rail) = (Distance)/(Speed\ of\ Sound\ in\ Rail)\\\\T_(rail) = (8.8\ m)/(5950\ m/s)\\\\T_(rail) = 0.00148\ s`

FOR THE WAVE TRAVELING THROUGH AIR:

`T_(air) = (Distance)/(Speed\ of\ Sound\ in\ Air)\\\\T_(air) = (8.8\ m)/(343\ m/s)\\\\T_(air) = 0.0256\ s`

The separation in time between two pulses can now be given as follows:

`\Delta T = T_(air)-T_(rail) \\\Delta T = 0.0256\ s - 0.00148\ s\\`

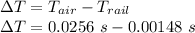
ΔT = 0.02412 s