Question

Una mujer de masa m está parada en el borde de una mesa giratoria horizontal de momento de inercia I y radio R. La mesa al principio está en reposo y tiene libertad de moverse alrededor de un eje vertical sin fricción que pasa por su centro. La mujer empieza a caminar alrededor de la orilla en direción de las manecillas del reloj (vista desde arriba) a una velocidad constante v en relación a la Tierra.

a) En qué dirección y con qué velocidad angular gira la mesa?

b) Cuál es el trabajo efectuado por la mujer para poner en movimiento la mesa?

Answer 1

r

`- (mR^2 )/(I ) \ v`Answer:

a) w = -
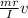`(m r )/(I) v` , b) W = - ½ m_woman R² (1 + m_woman R / I²) v²

Step-by-step explanation:

a) To solve this exercise, let's use the conservation of angular momentum.

We define a system formed by the table and the woman, therefore the torques are internal and the moment is conserved

initial instant. Before starting to move the woman

L₀ = 0

final instant. After starting to move

L_f = I w + m v r

the moment is preserved

L₀ = L_f

0 = Iw + m v r

w = -
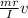`(m r )/(I) v` (1)

the direction of the angular velocity is opposite to the direction of the linear velocity, that is, counterclockwise

b) for this part we use the relationship between work and kinetic energy

W = ΔK

in this case the initial speed is zero and the final speed of the table, using the relationship between linear and angular variables

v = w r

we substitute

W = 0 - ½ I_total w²

I_total = I + m_{woman} R²

W = - ½ (I + m_woman R²) (
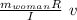`(m_(woman) R)/(I) \ v`) ²

W = - ½ (m_woman² R² + m_woman³ R³ / I²) v²

W = - ½ m_woman R² (1 + m_woman R / I²) v²