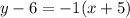Answer:
y - 6 = -1 (x+5)
Explanation:
1) First, find the slope of the line. Use the slope formula
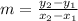 . Substitute the x and y values of (-5,6) and (0,1) into the formula and simplify like so:
. Substitute the x and y values of (-5,6) and (0,1) into the formula and simplify like so:
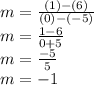
So, the slope of the line is -1.
2) Now we have enough information to write the equation of the line in point-slope form. Use the point-slope formula
 and substitute real values for the
and substitute real values for the
 ,
,
 , and
, and
 .
.
Since
 represents the slope of the line, substitute -1 in its place. Since
represents the slope of the line, substitute -1 in its place. Since
 and
and
 represent the x and y values of a point the line intersects, substitute the x and y values of (-5, 6) in those places as well. This gives the following equation and answer:
represent the x and y values of a point the line intersects, substitute the x and y values of (-5, 6) in those places as well. This gives the following equation and answer: