Answer:
650,000,000 student ID numbers are possible if the letters cannot be repeated.
Explanation:
The order in which the digits or letters are placed is important, which means that the permutations formula is used to solve this question.
Permutations formula:
The number of possible permutations of x elements from a set of n elements is given by the following formula:

In this question:
2 letters from a set of 26(permutations, as letters cannot be repeated).
6 digits, each with 10 possible outcomes.
How many student ID numbers are possible if the letters cannot be repeated?
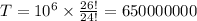
650,000,000 student ID numbers are possible if the letters cannot be repeated.