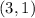Answer:

Explanation:
Given

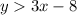
Required
Solve graphically
See attachment for graph;
The blue shade represents

While the green, represents
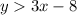
Next, determine the intersection points between the two lines
From the attached graph, we have:

Hence, the solution is: