Answer:
Explanation:
a. This is a decay function, since the final value (A) gets smaller and smaller as the days pass by.
b. The bacteria is decreasing by 75% per day exponentially.
c. In order to calculate the amount of bacteria in a week we simply substitute the variable t for 7 (7 days in a week) and solve for A
A = 200(0.75)^7
A = 26.7 bacteria after 1 week
d. In order to calculate this we need to substitute the variable A with 100 and solve for t
100 = 200(0.75)^t ... divide both side by 200
0.5 = 0.75^t
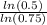 = t
= t
2.4 = t
It will be half the initial amount after 2.4 days.