Question

A uniform plank of mass 10kg and length 10m rests on two supports, A and B as shown. A boy of weight 500N stands at a distance of 2m from A. Find the reaction forces U¹ and U² at the supports A and B respectively​

Answer 1

U² = 142.86 N

U¹ = 357.14 N

Step-by-step explanation:

Taking summation of the moment about point A, we get the following equilibrium equation: (taking clockwise direction as positive)

`W(2\ m) - U^2(7\ m) = 0`

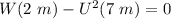
where,

W = weight of boy = 500 N

U² = reaction ay B = ?

Therefore,

`(500\ N)(2\ m)-(U^2)(7\ m)=0\\U^2=(1000\ Nm)/(7\ m)\\`

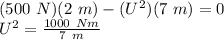
U² = 142.86 N

Now, taking summation of forces on the plank. Taking upward direction as positive, for equilibrium position:

`W-U^1-U^2=0\\500\ N - 142.86\ N = U^1\\`

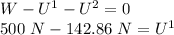
U¹ = 357.14 N