Answer:
(1664π)/7
Explanation:
We first need to know the points of intersection of the two functions, y = x³ and y = 4x.
To do this, we equate both functions
x³ = 4x
x³– 4x = 0
x(x² – 4) = 0
x(x – 2)(x + 2) = 0
x = 0, x = 2 and x = –2
Since we are looking for the volume formed by rotating the region inside the FIRST QUADRANT, then the needed points of x are 0 and 2. These are our limits of integration.
The formula for the area of the solid is
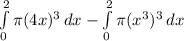
This gives:
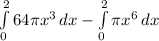
On integration, we have
16π(2⁴) – π(2^7)/7
=
256π – 128π/7
= (1792π – 128π)/7
= (1664π)/7