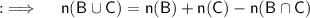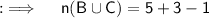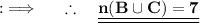Question

Let U = {English, French, History, Math, Physics, Chemistry, Psychology, Drama}, A = {Math, History, Chemistry, English}, B = {Psychology, Drama, French, Chemistry, English}, and C = {Physics, History, French}. Find the following. n(B u C)

Answer 1

We are provided with ;

• 
  `{\sf{U=\{English,\: French,\: History,\: Math,\: Physics,\: Chemistry,\: Psychology,\: Drama\}}}`
• 
  `{\sf{A=\{Math,\: History,\: Chemistry,\: English\}}}`
• 
  `{\sf{B=\{Psychology,\: Drama,\: French,\: Chemistry,\: English\}}}`
• 
  `{\sf{C=\{Physics,\: History,\: French\}}}`

And we need no find
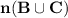`{\bf n(B\cup C)}` . At first Let me tell you that here
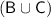`{\sf (B\cup C)}` represents the union of the set B and C.And
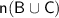`{\sf n(B\cup C)}` represents the cardinal no. of the respective set or no. of elements present in the respective set.So let's find the set
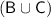`{\sf (B\cup C)}` first

`{:\implies \quad \sf B\cup C=\{Physics,\: Drama,\: French,\: Chemistry,\: English,\: Psychology,\:History\}}`

`{:\implies \quad \bf \therefore \quad \underline{\underline{n(B\cup C)=7}}}`

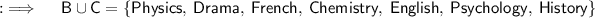
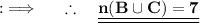
Alternative Method :-

As we know that ;

• 
  `{\boxed{\bf n(A\cup B)=n(A)+n(B)-n(A\cap B)}}`

Where
`{\sf (A\cap B)}` is the Intersection of A and B or the set of common elements of A and B. So now by same concept , now finding the intersection of B and C :-

`{:\implies \quad \sf B\cap C=\{French\}}`

So ,
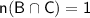`{\sf n(B\cap C)=1}` . Now , putting the values in the formula;

`{:\implies \quad \sf n(B\cup C)=n(B)+n(C)-n(B\cap C)}`

`{:\implies \quad \sf n(B\cup C)=5+3-1}`

`{:\implies \quad \bf \therefore \quad \underline{\underline{n(B\cup C)=7}}}`