Question

Sometimes a person cannot clearly see objects close up or far away. To correct this type of vision, bifocals are often used. The top half of the lens is used to view distant objects and the bottom half of the lens is used to view objects close to the eye. Bifocal lenses are used to correct his vision. A diverging lens is used in the top part of the lens to allow the person to clearly see distant objects.

1. What power lens (in diopters) should be used in the top half of the lens to allow her to clearly see distant objects?
2. What power lens (in diopters) should be used in the bottom half of the lens to allow him to clearly see objects 25 cm away?

Answer 1

1) P₁ = -2 D, 2) P₂ = 6 D

Step-by-step explanation:

for this exercise in geometric optics let's use the equation of the constructor

`(1)/(f) = (1)/(p) + (1)/(q)`

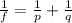
where f is the focal length, p and q are the distance to the object and the image, respectively

1) to see a distant object it must be at infinity (p = ∞)

`(1)/(f_1) = (1)/(q)`

q = f₁

2) for an object located at p = 25 cm

`(1)/(f_2) = (1)/(25) + (1)/(q)`

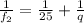
We can that in the two expressions we have the distance to the image, this is the distance where it can be seen clearly in general for a normal person is q = 50 cm

we substitute in the equations

1) f₁ = -50 cm

2)

`(1)/(f_2) = (1)/(25) + (1)/(50)`

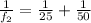
`(1)/(f_2)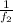` = 0.06

f₂ = 16.67 cm

the expression for the power of the lenses is

P =
`(1)/(f)`

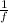
where the focal length is in meters

1) P₁ = 1/0.50

P₁ = -2 D

2) P₂ = 1 /0.16667

P₂ = 6 D