Answer:
The 90% confidence interval for p is (0.8236, 0.9564). The upper confidence limit for p is 0.9564.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the zscore that has a pvalue of
 .
.
He discovers that a particular weed killer is effective 89% of the time. Suppose that this estimate was based on a random sample of 60 applications.
This means that
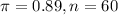
90% confidence level
So
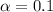 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
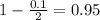 , so
, so
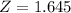 .
.
The lower limit of this interval is:
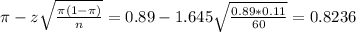
The upper limit of this interval is:
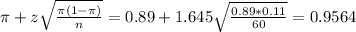
The 90% confidence interval for p is (0.8236, 0.9564). The upper confidence limit for p is 0.9564.