Final answer:
The volume V(x) of Mai's open-top box, created by cutting out corners with side length x from a 10 cm by 10 cm square piece of cardboard and folding up the sides, is V(x) = 4
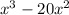 + 100x cubic centimeters.
+ 100x cubic centimeters.
Step-by-step explanation:
To determine the volume of Mai's open-top box, we start with a 10 cm by 10 cm square piece of cardboard. When a square of side length x is cut from each corner, and the sides are folded up, the new dimensions of the box will be:
Length: 10 - 2x cm
Width: 10 - 2x cm
Height: x cm
The volume V(x) of an open-top box can be calculated by multiplying these three dimensions, that is:
V(x) = (10 - 2x)(10 - 2x)x
Or more simply:
V(x) = x(100 - 20x + 4
 )
)
V(x) = 4
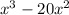 + 100x
+ 100x
So, the function representing the volume of the box V(x) as a function of the side length of the square cutouts x is:
V(x) =
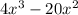 + 100x
+ 100x