Explanation:
To find the displacement I'll give you two ways since I don't know if you had experience with calculus. So here's both ways.
Way 1: Graph 1: Geometric Apporoach.
I'll a graph where t is x axis and v is y axis. So we have a velocity versus time graph.
Then, we graph the function
Look Above this what graph I get
Now, to find displacement,
Remeber displacement is the product of velocity and time. So here displacement is area under the curve.
We want displacement from 0 to 2. so let form the shape under the curve.
Look at the second graph, we form a triangle and we get this
Area of Triangle is 1/2 bh.
The base is 2 and the height is -6 so we get
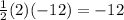
Our height is -12 because velocity is a vector so it can be negative and down is negative.
So the displacement is -12
Way 2: Calculus Way.
We can use integration to find the answer.
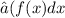
So now we have

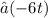
Now we do,

So the area under the curve is -12. Thus displacement is -12