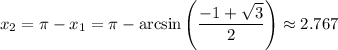Question

Please Help! Trigonometry Problem:
find the solution in 0< x < 2π
cos 2x = 2sinx

Answer 1

`\displaystyle x=\left\{\arcsin\left((-1+√(3))/(2)\right), \pi-\arcsin\left((-1+√(3))/(2)\right)\right\}`

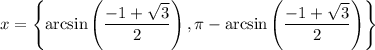
Or their approximations:

`x\approx \left\{0.375, 2.767\right\}`

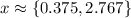
Explanation:

We are given:

`\cos(2x)=2\sin(x)`

And we want to find the solution in [0, 2π).

Recall the double-angle identities for cosine:

`\begin{aligned} \cos(2x)&=\cos^2(x)-\sin^2(x) \\&=2\cos^2(x)-1\\&=1-2\sin^2(x)\end{aligned}`

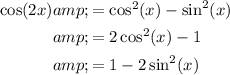
We will use the third version. Hence:

`1-2\sin^2(x)=2\sin(x)`

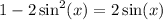
Move all terms to one side:

`-2\sin^2(x)-2\sin(x)+1=0`

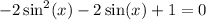
This is now in quadratic form. For simplicity, let u = sin(x):

`-2u^2-2u+1=0`

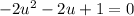
Solve for u. Simplify:

`2u^2+2u-1=0`

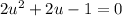
By the quadratic formula:

`\displaystyle u=(-(2)\pm√((2)^2-4(2)(-1)))/(2(2))}`

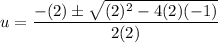
Evaluate:

`\displaystyle u=(-1+√(3))/(2)\approx 0.366\text{ and } u=(-1-√(3))/(2)\approx-1.366`

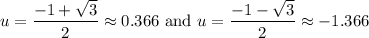
Note that the second solution is > -1. Hence, we will disregard it. (The range of sine is only -1 ≤ y ≤ 1.)

Back-substitute:

`\displaystyle \sin(x)=(-1+√(3))/(2)`

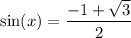
Since it is approximately 0.366, it will occur twice (once in QI and again in QII. This is because sine is positive only in those two quadrants). Using a calculator:

`\displaystyle x_1=\arcsin\left((-1+√(3))/(2)\right)\approx0.375`

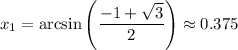
Using reference angles, the other solution is:

`\displaystyle x_2=\pi -x_1=\pi -\arcsin\left((-1+√(3))/(2)\right) \approx2.767`