9514 1404 393
Answer:
True
Explanation:
We assume your equation is intended to be ...
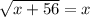
The usual method of solution squares both sides, then solves the resulting quadratic.
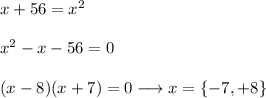
Considering the initial problem, we require x ≥ 0, so the x=-7 solution is extraneous.
__
A graphical solution shows there is only one value of x that satisfies the equation. The other "solution" arises from considering the negative square root. It is introduced when the equation is squared.