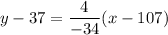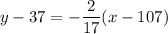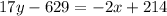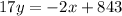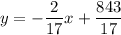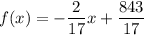Question

Elena notices that when she spends less time on social media the night before a quiz, she gets a higher score. Before one quiz, she spent 107 minutes on social media and eamed 37 points on a

quiz. Before another quiz, she spent 73 minutes on social media and eamed 11 points on a quiz
write a function to model a linear relationship between Elena's social media usage, in minutes, and her quiz scores, assuming that the total number of points on each quiz remains a constant
Respond in the space provided

Answer 1

`f(x) = -(2)/(17)x + (843)/(17)`

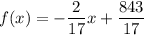
Explanation:

Given:

Score of 37 with 107 minutes on social media.

Score of 41 with 73 minutes on social media.

The two pieces of information above can be thought of as two points on a line. Since the quiz score is a function of the number of minutes on social medial, let the number of minutes on social media be x and the score be y. The given information gives us two ordered pairs: (107, 37) and (73, 41).

Now we need to write the equation of a line that passes through these two points.

The two-point form of the equation of a line is:

`y - y_1 = (y_2 - y_1)/(x_2 - x_1)(x - x_1)`

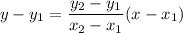
We have
`x_1 = 107`,
`y_1 = 37`,
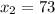`x_2 = 73`, and
`y_2 = 41`.

`y - 37 = (41 - 37)/(73 - 107)(x - 107)`

`y - 37 = (4)/(-34)(x - 107)`

`y - 37 = -(2)/(17)(x - 107)`

`17y - 629 = -2x + 214`

`17y = -2x + 843`

`y = -(2)/(17)x + (843)/(17)`

`f(x) = -(2)/(17)x + (843)/(17)`