Question

Korey kept track of the number of miles he ran each week for five weeks. The median number of miles he ran during the five weeks was 20, and the mean was 21. Which list could show the number of miles Korey ran each of the five weeks?

Answer 1

Options:

A. 18, 20, 20, 22, 25

B. 20, 20, 20, 25, 25

C. 16, 19, 21, 22, 22

D. 20, 20, 21, 22, 22

Answer:

A. 18, 20, 20, 22, 25

Explanation:

Required

Which list has a mean of 21 and median of 20

Each of the list have 5 numbers and they've all been sorted.

The median is the number at the 3rd position (i.e. the middle number)

So, list C and D are out because they do not have a median of 20.

Next, calculate the mean of lists A and B

`\bar x = (\sum x)/(n)`

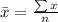
A. 18, 20, 20, 22, 25

`\bar x = (18 + 20 + 20 + 22 + 25)/(5)`

`\bar x = (105)/(5)`

`\bar x = 21`

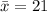
B. 20, 20, 20, 25, 25

`\bar x = (20 + 20 + 20 + 25 + 25)/(5)`

`\bar x = (110)/(5)`

`\bar x = 22`

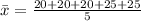
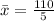
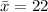
Only list A has a median value of 20 and a mean value of 21