Answer:
Yes it will be appropriate to model the distribution of a sample mean with a normal model
Explanation:
Given that the population is not normal, and the sample is sufficiently large, according to the Central Limit theorem, the distribution of the mean pf the sampling distribution will be approximately normal not withstanding the population from which the sample is obtained. Therefore, the mean,
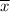 , and the standard deviation,
, and the standard deviation,
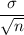 , of the sample will be equal to the mean, μ, and standard deviation, σ, of the of the population
, of the sample will be equal to the mean, μ, and standard deviation, σ, of the of the population
Therefore, it will be appropriate to model the distribution of a sample mean with a normal model