Question

If x = 1 does x² - x = 0?

Answer 1

Given,

x = 1

We need to find if x² - x = 0 is true or false.

If x = 1, then x² will be equal to 1² = 1 (1 multiplied by 1 is one / 1 square is equal to 1)

So,

=》x² - x = 0

By substituting ...

=》1² - 1 = 0

Now calculate...

=》1 - 1 = 0

=》0 = 0

•°• The given statement is true.

_____

RainbowSalt2222 ☔

Answer 2

Yes it does

Explanation:

we would like to Prove the following for x=1:

`\displaystyle {x}^(2) - x = 0`

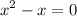
since x=1 substitute:

`\displaystyle {1}^(2) - 1 \stackrel {?}{=}0`

to simplify it we can consider the order of PEMDAS which is a abbreviation of

• Parentheses
• Exponent
• Multiplication or
• Division
• Addition or
• Substraction

since exponent come first

simplify exponent:

`\displaystyle {1}^{} - 1 \stackrel {?}{ = }0`

simplify substraction:

`\displaystyle 0 \stackrel { \checkmark}{ = }0`

since left hand side equal to right hand side

hence, Proven

Alternate way:

use a²-b²=(a+b)(a-b) to rewrite:

`\displaystyle (x + √(x) )(x - √(x) ) = 0`

since x=1 substitute:

`\displaystyle (1 + √(1) )( 1- √(1) ) \stackrel {?}{=} 0`

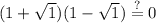
simplify square root:

`\displaystyle (1 + 1)( 1- 1 ) \stackrel {?}{=} 0`

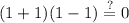
simplify parentheses:

`\displaystyle (2)( 0) \stackrel {?}{=} 0`

Multiplying any number by 0 results 0 thus

`\displaystyle 0 \stackrel { \checkmark}{=} 0`

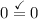
since left hand side equal to right hand side

hence, Proven