Answer:
The rocket will hit the ground after 113.28 seconds.
Explanation:
Solving a quadratic equation:
Given a second order polynomial expressed by the following equation:
 .
.
This polynomial has roots
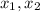 such that
such that
 , given by the following formulas:
, given by the following formulas:
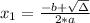

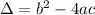
The height of a rocket, after t seconds, is given by:
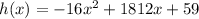
Using this equation, find the time that the rocket will hit the ground.
This is x for which
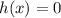 . So
. So
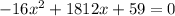
Then
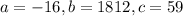

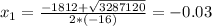
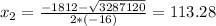
The rocket will hit the ground after 113.28 seconds.