Question

The depth of a river changes after a heavy rainstorm, Its depth, in feet, is modeled as a function of time, in hours. Consider this graph of the function. Enter the average rate of change for the depth of the river, measured as feet per hour, between hour 9 and hour 18. Round your answer to the nearest tenth

Answer 1

The average rate of change for the depth of the river measured as feet per hour is approximately 0.3 feet/hour

Explanation:

The depth of the river in feet with time is given by the function with the attached

From the graph, we have;

The depth of the river at hour t = 9 is f(9) = 18 feet

The depth of the river at hour t = 18 is f(18) = 21 feet

The average rate of change, A(x), for the depth of the river measured as feet per hour is given as follows;

`A(X) = (f(b) - f(a))/(b - a)`

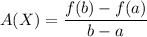
Therefore, for the river, we have;

`A(X) = (f(18) - f(9))/(18 - 9) = (21 - 18)/(18 -9) = (3)/(9) =(1)/(3)`

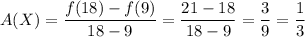
The average rate of change for the depth of the river measured as feet per hour A(X) = 1/3 feet/hour

By rounding the answer to the nearest tenth, we have;

A(X) = 0.3 feet/hour.