Answer:
The right solution is "$178.86".
Step-by-step explanation:
The given values are:
Interest rate,
= 10%
New nominal interest rate,
= 8%
Years,
= 24
As per the question,
On the original loan, the annul installments will be:
=
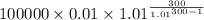
=
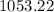 ($)
($)
As we know,
The remaining 156 instalments are charged throughout the PV after the 144th deposit,
=

=
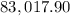 ($)
($)
On the refinanced loan, the annul installments will be:
=
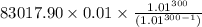
=
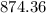 ($)
($)
hence,
After refinancing, the difference in mortgage will be:
=
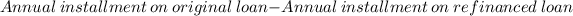
=
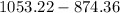
=
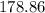 ($)
($)