Question

1.13 UNIT TEST GRAPH OF SINUSOIDAL FUNCTION PART 1

What is the equation of the midline for the function f(x)?

f(x) =1/2 sin(x)+6

Answer 1

The equation of the midline for the function
`f(x)` is
`y = 6`.

Explanation:

The sinusoidal function of the form
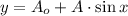`y = A_(o)+A\cdot \sin x` is a periodic function whose range is bounded between
`A_(o)-A` (minimum) and
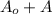`A_(o)+A` (maximum). The equation of the midline is a line paralel to the x-axis, that is:

`y = c,\forall\, c\in \mathbb{R}` (1)

Where
`c` is mean of the upper and lower bounds of the sinusoidal function, that is:

`c = ((A_(o)+A+A_(o)-A))/(2)`

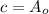`c = A_(o)` (2)

If we know that
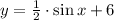`y = (1)/(2)\cdot \sin x + 6`, then the equation of the midline for the function
`y` is:

`c = A_(0) = 6`

`y = 6`

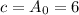
The equation of the midline for the function
`f(x)` is
`y = 6`.