Question

15 POINTS! PLEASE HELP!

To measure the distance across a wide river surveyors use a technique of measuring angles to a fixed point on the other side of the river. In the diagram below, a survey starts a point A and find m

Answer 1

DC = 1332.95 feet

Explanation:

From the figure attached,

Let the measure of DC = h ft

And measure of side BC = a ft

By applying tangent ratio in ΔBCD,

tan(56°) =
`\frac{\text{Opposite side}}{\text{Adjacent side}}`

=
`(DC)/(BC)`

=
`(h)/(a)`

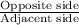
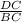
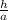
h = a[tan(56°)] --------(1)

By applying tangent rule in ΔACD,

tan(22°) =
`\frac{\text{Opposite side}}{\text{Adjacent side}}`

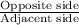
tan(22°) =
`(DC)/(BC+AC)`

=
`(h)/(a+2400)`

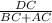
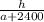
h = (a + 2400)tan(22°) ------- (2)

Equating the values of h from equations (1) and (2),

a[tan(56°)] = (a + 2400)tan(22°)

a[tan(56°) - tan(22°)] = 2400[tan(22°)]

1.0785a = 968.6629

a = 899.085 ft

From equation (1),

h = 899.085[tan(56°)]

h = 1332.948

h ≈ 1332.95 ft