Question

chord AB is 3cm from the center of the circle, the radius of the circle = 5cm,calculate the length of the chord​

Answer 1

The lenght of the chord is 8 cm.

Explanation:

The chord AB is 3 cm from the center of the circle. If we visualize the chord (in a horizontal position) and the radius of the circle (in a diagonal position) we can notice that both of them forms a triangle, with the following dimentions:

b: is the base =?

s: is one side of the triangle = distance of the chord from the center of the circle = 3 cm

h: is the hipotenuse = radius of the circle = 5 cm

To find the base (or the ohter side of the triangle) we need to use Pitagoras:

`b = \sqrt{h^(2) - s^(2)} = \sqrt{(5 cm)^(2) - (3 cm)^(2)} = 4 cm`

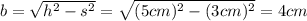
The above value is the half of the chord AB, so:

`\overline{AB} = 4cm*2 = 8 cm`

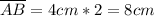
Therefore, the lenght of the chord is 8 cm.

I hope it helps you!