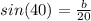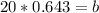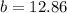Answer:
Explanation:
The sum of the three angles in a triangle is
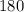 .
.
We know that a right angle is
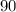 , and we are given another angle that is
, and we are given another angle that is
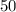 , so that means that angle
, so that means that angle
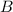 is:
is:
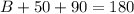
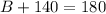
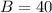
To find side length
 , we can use the
, we can use the
 function:
function:
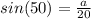
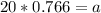

To find side length
 , we can again use the
, we can again use the
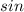 function:
function: