Answer:
 ,
,
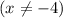
Explanation:
Given,
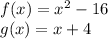
Find,
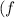 ÷
÷
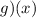
1. Approach
The easiest way to solve the problem is to set up the problem as a fraction. Then factor both the numerator (value on top of the fraction bar), and the denominator (value under the fraction bar). After doing so, simplify the fraction. Remember to take note of the value eliminated from the denominator, for this value will serve as the domain of the expression.
2. Divide the two functions
The two given functions are the following:
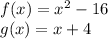
Set up the division problem,
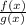
Substitute,
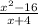
Factor,
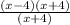
Simplify,

3. Find the domain of the expression
The value that was removed from the denominator is the following:
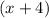
Set this equal to zero and solve,
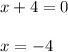
This expression is true as long as (
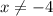 ).
).