Answer:
a. mean = 1000
standard deviation = 4358.9
b. expected value of average damage bar Y = 1000
probability bar y exceeds 2000 = 0.011
Explanation:
we have p1 = 95%, y1 = 0, p2 = 5%, y2 = 20000
Mean = (0.95 * 0) + (0.05 * 20000)
= 1000
var(y) = E(y²) - E(Y)²
= we solve for E(y)²
= 0²*0.95 + 20000²*0.05
= 0 + 20000000
then the variance of y = 20000000 - 1000²
=20000000-1000000
= $19000000
standard deviation is the square root of variance
= √19000000
= 4358.9
2.
a. Expected value of average is also the mean = 1000
b. we are to find probability that barY exceeds 2000
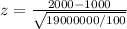
= 1000/435.889
= 2.29
1-p(z≤2.29)
= 1 - 0.989
= 0.011
so the probability that barY exceeds 2000 is 0.011