Question

Find the area of each regular polygon. Round your answer to the nearest tenth if necessary​

Answer 1

Explanation:

13). Area of a square = (Side)²

= (BC)²

Since, diagonals of a square bisect each other at 90°,

ΔBOC is a right triangle.

By applying Pythagoras theorem in the given triangle,

BC² = OB² + OC²

BC² = 2(OB)²

BC² = 2(7√2)²

BC =
`√(196)`

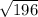
Area of square ABCD = (BC)²

= (√196)²

= 196 units²

14). Measure of interior angles of the regular hexagon = 120°

Area of the regular hexagon =
`(3√(3))/(2)(\text{side})^2`

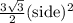
From the given picture,

m∠BAC = m∠ABC = m∠ACB = 60°

Therefore, ΔABC is an isosceles triangle.

And all sides of this triangle will be equal in measure.

AB = AC = BC = 9 units

Area of the given regular hexagon =
`(3√(3))/(2)(9)^2`

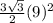
= 210.44 square units

≈ 210.4 square units