Answer:

Explanation:
Linear equations are typically organized in slope-intercept form:
 where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)
where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)
1) Determine the slope (m)
Parallel lines will always have the same slope. Therefore, this line will have the same slope as the given line
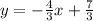 .
.
Plug in
 as the slope
as the slope
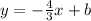
2) Determine the y-intercept (b)
To find the y-intercept, plug the given point (-5,2) into the equation and solve for b.
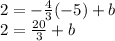
Subtract both sides by
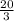
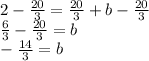
Therefore, the y-intercept is
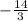 .
.
3) Plug the y-intercept back into our original equation

I hope this helps!