Given:
A line passes through the points (9,9) and
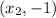 .
.
The slope of the line is
 .
.
To find:
The value of
![x_2]](https://img.qammunity.org/2022/formulas/mathematics/high-school/hynt1ads672rjk67rnemph80p2tkj2r5j6.png) .
.
Solution:
Slope formula:

The line passes through the points (9,9) and
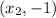 . So, the slope of the line is:
. So, the slope of the line is:
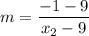
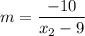
It is given that the slope of the line is
 .
.
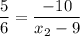
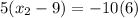
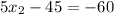


Divide both sides by 5.
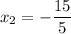

Therefore, the value of
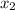 is
is
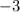 .
.