Answer:
The statement which explains how the total time spent in the air is affected as the projectile's angle of launch increases from 25 degrees to 50 degrees is;
C. Increasing the angle from 25° to 50° will increase the total time spent in the air
Step-by-step explanation:
The equation that can be used to find the total time, T, spent in the air of a projectile is given as follows;
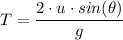
Where;
T = The time of flight of the projectile = The time spent in the air
u = The initial velocity of the projectile
θ = The angle of launch of the projectile
g = The acceleration due to gravity ≈ 9.81 m/s²
Given that sin(50°) > sin(25°), when the angle of launch, θ, is increased from 25 degrees to 50 degrees, we have;
Let T₁ represent the time spent in the air when the angle of launch is 25°, and let T₂ represent the time spent in the air when the angle of launch is 50°, we have;
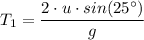
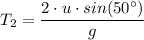
sin(50°) > sin(25°), therefore, we have;

Therefore;
T₂ > T₁
Therefore, increasing the angle at which the projectile is launched from 25° to 50° will increase the total time spent in the air.