Question

A manufacturer of banana chips would like to know whether its bag filling machine works correctly at the 421 gram setting. It is believed that the machine is underfilling the bags. A 26 bag sample had a mean of 413 grams with a standard deviation of 24. Assume the population is normally distributed. A level of significance of 0.02 will be used. Find the P-value of the test statistic. You may write the P-value as a range using interval notation, or as a decimal value rounded to four decimal places.

Answer 1

The p-value is approximately 0.0508

Explanation:

The given parameters are;

The expected mass of banana chips bag filled by the machine, μ = 421 gams

The number of bags in the sample of bags filled by the machine, n = 26 bags

The mean mass of the bags in the sample,
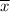`\overline x` = 413 grams

The standard deviation of the mass in the bags, s = 24 grams

The level of significance, α = 0.02

The null hypothesis, H₀; μ = 421 grams

The alternative hypothesis, Hₐ; μ < 421 grams

The test statistic is given as follows;

`t=\frac{\bar{x}-\mu }{(s)/(√(n))}`

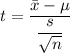
We get;

`t=(413-421 )/((24)/(√(26))) \approx -1.6996731712`

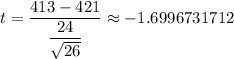
The t-value = -1.6996731712

The degrees of freedom df = n - 1 = 26 - 1 = 25

From an online source, we get the critical-t = 2.166587

The p-value is obtained using an online source as p(t ≤ -1.6996731712) = 0.0508

From the p-value table, we get

0.05 < p < 0.1