Answer:
f'₁ = 149.985 10⁶ Hz, f'₂ = 150.0149 10⁶ Hz
Step-by-step explanation:
We can solve this problem using the relativistic doppler effect
f’= f₀
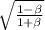
β = v/c
where the velocity is positive for the emitter and the receiver are moving away
In this case we must calculate the speed of the Earth, they tell us to consider the orbit as circular
v = w r
the angular velocity is related to the period
w = 2π / T
we substitute
v = 2π r / T
Let's reduce the period to the SI system
T = 1 year (365day / 1 year) (24h / 1day) (3600s / 1h) = 3.15 10⁷ s
we substitute
v = 2π 1.496 10¹¹ / 3.145 10⁷
v = 2.989 10⁴ m / s
We have two maximum possibilities when the planets approach and when the planets move away
the change of the emitted frequency
β = 2.989 10⁴/3 10⁸
β = 0.99625 10⁻⁴
we substitute in the equation to find the two frequencies
f’= f₀
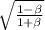
planets move away
f’₁ = 150 10⁶

f’₁ = 150 10⁶
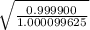
f’₁ = 1.50 10⁶ 0.999900
f'₁ = 149.985 10⁶ Hz
planets approaching
f’₂ = 150 10⁶

f’₂ = 150 10⁶ 1.0000996
f'₂ = 150.0149 10⁶ Hz
we see that the changes in the frequencies are extremely small