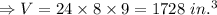Answer:
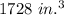
Explanation:
Given
The dimension of the rectangular base aquarium is
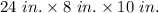
The gap between top and water level is
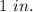 i.e. water is filled up to a height of
i.e. water is filled up to a height of
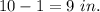
Now, the dimensions of the water in the aquarium is
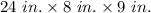
The volume of a rectangular base prism is
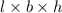
The volume of water in the aquarium is