Answer:
a = 54
b = 18
Explanation:
ABCD is a square. It's diagonals AC and BD intersects at G.
Since, diagonals of a square bisects at right angles.
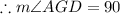
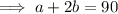
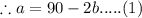
Since, measure of each angle of a square is right angle.
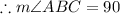
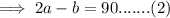
From equations (1) & (2)
2(90° - 2b) - b = 90°
180° - 4b - b = 90°
-5b = 90° - 180°
-5b = - 90°
b = - 90°/(-5)
b = 18
Plug b = 18 in equation (1)
a = 90° - 2*18
a = 90° - 36°
a = 54