Answer:
Based on the given data, there is no evidence to support the claim that the standard deviation of the heights of women aged 25 to 34 have a different standard deviation from men aged 25 to 34
Explanation:
The standard deviation for the heights of men aged 25 to 34, σ₀ = 2.9
The population variance, σ₀ = 2.9² = 8.41
The significance level of the test = 0.05
The number of randomly selected women in the sample, n = 16
The given heights of the 16 randomly selected women are listed as follows;
62.13, 65.09, 64.18, 66.72, 61.15, 67.50, 64.65, 63.09, 63.80, 64.21, 60.17, 68.28, 66.49, 62.10, 65.73, 64.72
By using the Mean (Average) and standard deviation of a sample formula from Microsoft Excel, we have;
The mean height of the women,
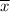 = 63.37563
= 63.37563
The standard deviation of the variance, s₂ = 2.2 78511
The null hypothesis, H₀; σ = 2.9
The alternative hypothesis, H₀; σ ≠ 2.9
The chi-square test is given as follows;
T = (N - 1)(s/σ₀)²
Therefore, we have;
T = (16 - 1)(2.278511/2.9)² ≈ 9.2597
The critical value for the lower tail = 6.908
The critical value for the upper tail = 28.845
For the critical region, we have;
Reject H₀ if T <6.908 or T > 28.845
Therefore, given that the critical T is larger than 6.908 and less than 28.845, we fail to reject the null hypothesis, and there is not enough statistical evidence to prove that there is a difference in the standard deviation of the heights of men and women aged 25 to 34