Answer:
The vertex is at
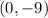
The axis of symmetry
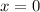
Explanation:
Note: You can answer all the questions looking at the graph
We have the function
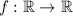 (I am assuming this) such that
(I am assuming this) such that
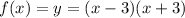
We note that
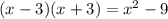 once we have a difference of squares
once we have a difference of squares
Therefore, we have a quadratic function.
So
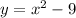
The vertex
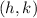 is
is
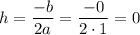
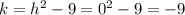
Therefore, the vertex is at
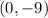
As we know the vertex, we conclude that the axis of symmetry
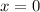
The x-intercepts occur at
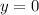
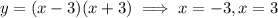
So, the left x-intercept is
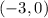 and the right x-intercept is
and the right x-intercept is
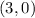
As I stated in the beginning, the domain is the set of all real numbers. Precisely,
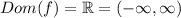
Once, we know the vertex, we also conclude that the Range is
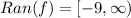
Greater than or equal to -9