Answer:

Explanation:
we are given a expression
we are said to solve it using L'Hôpital's Rule
recall, L'Hôpital's Rule:
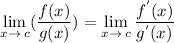
it is to say the ' means derivative
our given expression:

let's apply L'Hôpital's Rule
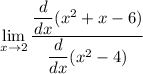
some formulas of derivative

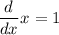
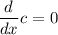
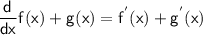
use sum derivative formula to simplify:
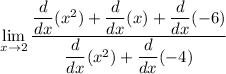
simplify using exponents using exponent derivative formula:

use variable derivative formula to simplify variable:
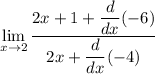
use constant derivative formula to simplify derivative:
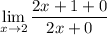
simplify addition:

since we are approaching x to 2
we can substitute 2 for x
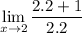
simplify multiplication:
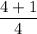
simplify addition:

hence,
